Algorithms
Contents
- Algorithm 1: Single Point Analysis
- Algorithm 2: Multiple Point Analysis
- Algorithm 3: Basin of Attraction
- Algorithm 4: Phase Portrait
Single Point Analysis
The system of ODEs, $F(\tilde{x}(t))$ are solved algebraically to find the fixed-point solutions, $\tilde{x}^{g,\ast}$, where $g$ represents the number of fixed-points found. $\tilde{x}$ is the vector of variables ($X$ and $S$) associated with the motifs. Two methods are available for analysis of the stability of the fixed-points; Linear Stability Analysis and the Routh-Hurwitz criterion. In the former, $\epsilon$ defines the tolerance threshold for stability.

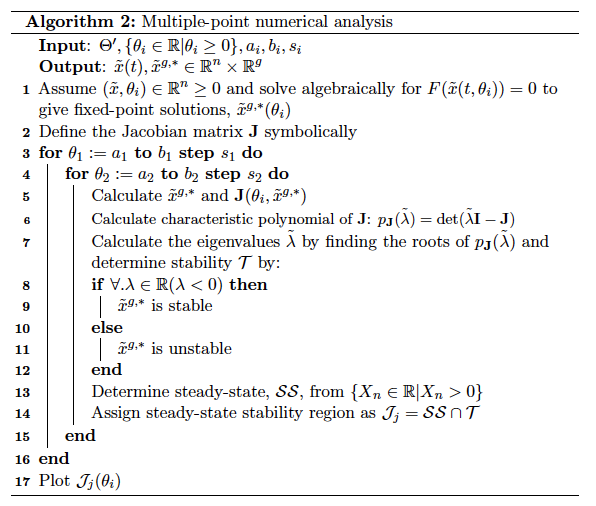
Multiple Point Analysis
This is an extension of the Single Point Analysis, extended for multiple points ($s_i$) within the range ($a_i$,$b_i$) for a pair of parameters ($\theta_{i}$), where $i = (1,2)$. In biological terms, the chosen parameters may represent operational properties of the system (e.g., dilution rate $D$, substrate input concentration $S_{in}$) or of the organisms themselves (e.g., growth rates, substrate yields), and thus can be used to test the effect of these parameters on the behaviour of the system itself.
Basin of Attraction

Phase Portrait
